Shrink Indecomposable Fractals

Sigrid Ewert (Department of Computer Science, University of Stellenbosch, South Africa)

Andries van der Walt (Department of Mathematics, University of Stellenbosch, South Africa)

Abstract:

Iterated Function Systems (IFSs) are among the best­known methods for constructing fractals. The sequence of pictures E₀ , E₁ , E₂ , ... generated by an IFS {X; f₁ , f₂ , ... , f_t } converges to a unique limit 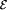, which is independent of the choice of starting set E₀, but completely determined by the choice of the maps f_i .

Random context picture grammars (rcpgs) are a method of syntactic picture generation. The terminals are subsets of the Euclidean plane and the replacement of variables involves the building of functions that will eventually be applied to terminals. Context is used to enable or inhibit production rules.

We show that every IFS can be simulated by an rcpg that uses inhibiting context only. Since rcpgs use context to control the sequence in which functions are applied, they can generate a wider range of fractals or, more generally, pictures than IFSs. We give an example of such a fractal. Then we show that under certain conditions the sequence of pictures generated by an rcpg converges to a unique limit.

Categories: F.4.2