Integrated Applications of Electronic Structure
Computations in the Undergraduate
Chemistry Curriculum
Rita K. Hessley
(The University of Cincinnati, Cincinnati, OH
rita.hessley@uc.edu)
Daniel L. Morris, Jr.
(Rose-Hulman Institute of Technology, Terre Haute, IN
daniel.morris@rose-hulman.edu)
Michael R. Mueller
(Rose-Hulman Institute of Technology, Terre Haute, IN
michael.mueller@rose-hulman.edu)
Abstract: This paper describes the integrated use of electronic
structure computations in the undergraduate chemistry curriculum including
organic, physical, and analytical chemistry courses. This computational
tool is used to enhance student learning and understanding of chemical
principles along with exposing students to a modern research tool in chemistry.
The electronic structure computations are used for homework, classroom
activities (including examinations), and laboratory experiments (both computational
and wet-lab). Some examples of these uses of electronic structure computations
in organic, analytical, and physical chemistry courses are discussed.
Key Words: Academic Computing, Chemistry, Electronic Structure
Computations, Undergraduate Chemistry Curriculum
Category: J
1 Introduction
The use of electronic structure computations in chemistry is not novel.
The study of chemistry is based upon the fact that chemical behavior is
dependent upon structure, especially electronic structure. Therefore, the
behavior of a particular chemical species can be predicted on the basis
of its structure. Molecular electronic computations have become an important
aspect of both experimental and theoretical chemical research. Computational
chemistry is fast becoming a tool as important as nuclear magnetic resonance
(NMR) spectrometry. just as no one would argue the importance of including
the theory, use, and interpretation of NMR in the undergraduate chemistry
curriculum, computational chemistry, especially molecular electronic computations,
has become just as important.
As computers became powerful enough to handle large structures, chemists
began refining calculations in order to make accurate predictions about
the chemical properties of reactants and products. This is becoming increasingly
important in the ability to inhibit or promote biological reactions based
upon molecular recognition. It is also extremely important to be able to
predict potential drug activity and bioavailability in the area of pharmaceuticals.
Therefore, being able to make accurate predictions about the products of
specific reactions and the medicinal potential of a target product is sociologically
and economically desirable.
The use of computers has made a drastic change in teaching and learning
from the primary grades all the way into college. Most freshmen enter our
institutions of higher learning with a high degree of computer literacy.
It is becoming common for educational institutions to require that incoming
students purchase a laptop computer that will be used for their academic
careers. Those institutions that do not require such a purchase are under
pressure to provide computer facilities adequate for constant student use.
Given the increasing importance of computational chemistry, the availability
of computers in the academic setting, and reasonably priced electronic
structure computational software, it seems both appropriate and necessary
that we in academia employ such programs in the undergraduate chemistry
curriculum. The most obvious reason for using such computational programs
in the classroom is the thesis that students learn and retain by seeing
and doing. Perhaps the best scenario is for students to be able to build
molecules and perform computations at their own seats in the classroom
along with an instructor. Analyzing the data allows students to discover
for themselves the concept being presented, and the instructor can confirm
the conclusion and offer more detailed explanations as to why and how.
The second reason for incorporating electronic structure computations in
the undergraduate chemistry curriculum is to provide students with "hands-on"
experience using such programs. Clearly, computational chemistry is becoming
increasingly important in the corporate and academic worlds, and the professionals
of tomorrow can benefit from exposure to these tools today.
The purpose of this paper is to provide examples of how we have integrated
use of an electronic structure computational program into the undergraduate
chemistry curriculum in an institution that requires all incoming students
to purchase a laptop computer with a standard software suite. The use of
such a program in an undergraduate organic chemistry course sequence is
obvious because such courses are based on the fact that chemical behavior
and reactivity arise from molecular structure and conformation.
However, organic chemistry is not the only course sequence that finds
electronic structure computations a valuable instructional tool. Computational
programs are capable of calculating thermodynamic properties and geometries
of molecules, complexes, and radicals. Molecular parameters such as dipole
moments, vibrational frequencies, and vibrational modes can be used to
interpret and predict spectra. And, computed electron densities of molecules
can be used to explain the mechanistic details of chemical reactions from
a modern quantum mechanical perspective rather than relying on traditional
classical mechanical spring models or "memorized" group functionalities.
We suggest that these capabilities can be utilized in courses throughout
the undergraduate curriculum, and we present examples of how we have used
electronic structure computations in organic, physical, and analytical chemistry
courses. The examples we present arise from using PC-Spartan+
[Wavefunction, Inc.] in an undergraduate chemistry curriculum
composed of chemistry and chemical engineering majors with individual copies
of the program installed on their laptop computers. We remind the reader
that there is other comparable computational software on the market, and
we do not intend to recommend this specific package over any other.
2 Organic Chemistry
Organic chemistry is generally the second course sequence for students
majoring in chemistry or chemical engineering. In some programs it is taken
in the second term of the first year. In others it is taken in the second
year simultaneously with the first course in analytical chemistry. Regardless
of the timing, however, many students experience organic chemistry as radically
different from any of their prior learning experiences. One reason is that
it is seldom related more than superficially to the chemistry students
studied in high school. Another reason why so many students find this a
particularly challenging course is that it demands a higher degree of chemical
intuition than the general chemistry sequence. Conceptually organic chemistry
is not as mathematical or quantitative as the introductory course. And,
while competency in critical thinking is one of the overall goals most
educators seek to inculcate in their students by the time the students'
college careers end, at the Sophomore level, students are, on average,
not mature enough to wrestle effectively with the abstract critical thinking
demanded by organic chemistry. Over the years a variety of tools have been
developed to help students meet this challenge. Hand-held models rank near
the top of the list for ease of manipulation and for providing reasonably
accurate 3-dimensional spatial information about molecules. It seems apparent
from the rapid incursion of molecular modeling programs into instructional
settings that what has been lacking in such common tools, at least for
organic chemistry, is far more than the colorful 3-dimensional animation
the electronic medium provides. Even for molecular structure and modeling
software that is least sophisticated in its underlying mathematical complexity,
the appeal and the intrinsic pedagogical value lies in the presentation
of fundamental physical-chemical principles designed in such a way as to
visually display readily recognizable atomic/electronic features about
the structure from which students can make concrete connections to the
abstract principles associated with the properties and reactivity of that
structure. By frequently encountering the same or similar connections,
students can more rapidly learn to make credible, critically developed
predictions about properties and reactivity of molecules or in situations
or systems for which they have never had an example `explained'
to them.
2.1 Fundamentals of Structure-Reactivity Relationships
From their first courses in chemistry, all students have at least a
rudimentary knowledge of acids as proton donors. Thus, a simple computational
exercise they can carry out the first time they use modeling software is
one in which they construct a set
of related organic protic acids and model electronic charge density
associated with the acid proton. The visual displays are valuable for revealing
the impact of changing electron density as structure changes. These data
are plotted as a function of literature pKa values (provided
or student-searched), and with a high degree of certainty students
can predict either the pKa or the electronic charge for additional
structures in the set. Equally as important as exhibiting the predictive
ability of these computations, students can be assisted to focus on the
fundamental concept of the relationship of structure at the molecular level
to the property displayed at the macroscopic level. Other introductory
exercises are available in many publications [see Hehre
et al. 1996]. They involve both physical-structural and electro-magnetic
considerations. These activities have utility either as in-class activities
culminating in discussion of the unifying principles, or as out-of-class
assignments that make students responsible for bringing their own data
into class where they must share their understanding as part of the class
discussion.
2.2 Experimental Design and Formulation of Testable Hypotheses
We have used two other somewhat less usual pedagogical applications
of the computational features of modeling software to support the laboratory
portion of the organic chemistry course. One is to have students use the
computational information to plan the experimental design of the laboratory
experiment. The second is to use modeling data as the entrée for
instructing students in the skill of articulating a hypothesis, then critiquing
the hypothesis once the experimental data are in hand. Tied together, these
features provide the instructor with a two-dimensional approach to instruction.
First, it moves beyond many published exercises that serve to illustrate
reactions that are not practical for students in an academic laboratory.
Second, this approach is an attempt to replace entirely the use of predefined-outcomes,
the so-called verification laboratory exercise, with guided, but open-ended
laboratory experiences.
For one of these applications we have added a computational exercise
as a precursor to a more traditional experiment involving thin layer chromatography
(TLC). In the stand-alone experiment a set of related compounds is ordered
according to dipole moment by carrying out a specified sequence of paired
TLC elutions. We do not provide the dipole moment data or
the specified sequence. Rather, we discuss some of the theory of solvent-solute
interactions, describe the overall intent, and provide some guidelines
for the experiment. Students must extract the relevant dipole moment information
from modeling computations. Using their own data and their understanding
of the theory, students formulate a hypothesis about how the paired elutions
can be done efficiently to illustrate the theory. Prior to going into the
lab some time is devoted to a class discussion of the various plans. This
gives the class an opportunity to hear how others have approached the problem,
particularly in terms of an efficient use of time, and to hear the kinds
of affirmations or questions the instructor raises about the various ideas.
The amount of pre-lab or class time spent on this discussion can be varied
according to individual situations, but we have discovered that such discussion
does assist some students in seeing that there are some "easier"
and some "harder", more cumbersome, ways of getting this work
done.
Whether they collaborate on a plan or not, students are responsible
for articulating their own written hypothesis, describing the logic of
the sequence they use, carrying out the experiment, and critiquing the
hypothesis and limitations of the experiment against their own results.
Because the hypothesis must be reported before they carry out the experiment,
students cannot "work backward", making their hypothesis "look
good" when they know what the outcome is. Their critique is not expected
to be self-deprecating, but students are asked to deal with revealed gaps
in their thinking. An additional feature of this exercise is that the concepts
and the conclusions related to TLC are reiterated in a slightly different
context when students study gas chromatography and liquid chromatography
in analytical chemistry (vide infra).
2.3 Transition States and Reaction Mechanisms
More complex applications of electronic structure computations have
been devised for the latter part of a year's course. From about mid-way
through a first course in organic chemistry references to reaction mechanism,
transition state structures, and the interplay of thermodynamic control
and kinetic control are frequent. By this time in the course students are
thoroughly familiar with such things as the relative structure-stability
relationships of primary, secondary and tertiary species, strong and weak
nucleophiles, nucleophile-electrophile interactions, the relationship between
energy and stability, and the disjunction between thermodynamic
stability and rate. They are also capable of utilizing the software fully.
Computations for such structural features as electron-rich and electron-poor
reaction sites, the way in which bond formation and bond breaking can affect
transition state structure, and the thermodynamic parameters associated
with these structures can be incorporated into guided discussions of almost
any common synthetic organic laboratory exercise. What this permits is,
again, moving away from verification; i.e., not providing students
with the identity of all species nor all the steps in a given reaction,
but letting them explore, discover and propose some of these pieces for
themselves. This can be done by referring students to only the general
nature of the reaction then asking them to model the structures of reactants
and potential products and to use molecular geometry and electron density
to predict the reactive sites. From this they can move to the construction
of one or two possible transition states, computing the energy demanded
to attain such a structure, and then reflecting on what the relative energy
values convey about the relative ease with which such a structure would
form. While focusing on thermodynamics, students need to be urged to keep
in mind the absence in modeling of kinetics information,
and they can be asked to think about ways in which they might be able to
test reaction rates. Finally, the discussion can move to using all their
information, both thermodynamic and kinetic and including the limitations
they have recognized, to form a hypothesis about the product they think
they will isolate when they carry out the reaction under consideration.
What this approach "costs" in time and in terms of perhaps doing
fewer experiments of the verification type is, we believe, more than compensated
for in what it "pays" in dividends gained in breadth and depth
of experience and understanding.
3 Physical Chemistry
The physical chemistry curriculum can be divided into the following
major segments: thermodynamics and thermochemistry, chemical kinetics,
and quantum chemistry. Within this section, applications of molecular electronic
computations in each of these major areas will be described. However, the
applications presented here are not implied to be all-inclusive. The possibilities
are limitless.
3.1 Quantum Chemistry
When using most electronic structure computational packages, the user
is faced with a number of different computational approaches: molecular
mechanics, ab-initio, semi-empirical, and density functional. An important
focus in an undergraduate physical chemistry course is to introduce the
differences in the approaches amongst these various computational methods
along with the inherent advantages and disadvantages of each. As an example,
if structure is the desired outcome, all of the approaches provide reasonably
accurate results. Hence using the fastest computational approach (i.e.
molecular mechanics) is superior. If thermochemical comparisons are needed
between different molecules or reaction pathways, the semi-empirical approach
is the most computationally economical. These various examples lead to
different computational strategies that make using the computational tools
more effective. Using electronic structure computations in instructing
quantum chemistry makes the subject come alive for students and helps them
see the practical importance of quantum chemistry in every field of chemistry.
3.2 Thermochemistry
An important topic in physical chemistry is the ability to obtain thermochemical
information on unstable species or reaction intermediates and transition
state structures. Here is where electronic structure computational methods
are most certainly indispensable. Students learn how to construct intermediate
structures and perform computations. In addition, students can use fundamental
information such as rotational, vibrational, and electronic energies to
obtain various thermochemical properties such as heats of formation, total
energy, entropy, and free energy via statistical thermodynamic computations.
For these computations, a student obtains the molecular information from
the electronic structure computation and then uses suitable spreadsheet
software to perform the various statistical thermodynamic computations.
This assignment not only provides students with a useful research skill,
but it helps students understand thermodynamic principles from a molecular
level.
3.3 Chemical Kinetics
An excellent example of using electronic structure computations is to
have students construct a potential energy diagram along a reaction pathway.
A specific example is the elementary gas-phase reaction of H + F2
 HF + F. Students initially build a structure of F-F - H whereby the distance
between the fluorine atoms is the normal bond length of a fluorine molecule
and the distance between the fluorine and the hydrogen atom is essentially
infinite (i.e. in the order of 3 - 4 Angstroms). These distances between
the atoms along with angles (180°) are constrained, and the energy of this
structure is calculated. The Hamiltonian employed in the calculation depends
on the computational platform available to the students. Depending on the
Hamiltonian employed, the computation can become quite lengthy. Additional
points are obtained along the reaction pathway by increasing the distance
between the fluorine atoms and decreasing the distance between the hydrogen
and fluorine atoms. At each point, the distances and angles between the
atoms are constrained and the energy obtained. Students obtain numerous
energy points along the reaction pathway from which a three dimensional
plot can be constructed: the F-F distance verses the H-F distance verses
energy. (Suitable programs for constructing 3-D plots include algebraic
software such as Maple [Waterloo Maple Inc.].)
From this plot, students can identify the transition state structure along
with the activation energy of this reaction. Since the computational activity
is quite involved, it is helpful to make this into a group assignment (groups
composed of three to four students) so the computational effort can be
shared. These computations can be further manipulated through animation
features that help students visualize vibrational modes that lead successfully
to products.
HF + F. Students initially build a structure of F-F - H whereby the distance
between the fluorine atoms is the normal bond length of a fluorine molecule
and the distance between the fluorine and the hydrogen atom is essentially
infinite (i.e. in the order of 3 - 4 Angstroms). These distances between
the atoms along with angles (180°) are constrained, and the energy of this
structure is calculated. The Hamiltonian employed in the calculation depends
on the computational platform available to the students. Depending on the
Hamiltonian employed, the computation can become quite lengthy. Additional
points are obtained along the reaction pathway by increasing the distance
between the fluorine atoms and decreasing the distance between the hydrogen
and fluorine atoms. At each point, the distances and angles between the
atoms are constrained and the energy obtained. Students obtain numerous
energy points along the reaction pathway from which a three dimensional
plot can be constructed: the F-F distance verses the H-F distance verses
energy. (Suitable programs for constructing 3-D plots include algebraic
software such as Maple [Waterloo Maple Inc.].)
From this plot, students can identify the transition state structure along
with the activation energy of this reaction. Since the computational activity
is quite involved, it is helpful to make this into a group assignment (groups
composed of three to four students) so the computational effort can be
shared. These computations can be further manipulated through animation
features that help students visualize vibrational modes that lead successfully
to products.
The applications of electronic structure computations to undergraduate
physical chemistry courses are endless. Not only is incorporation of these
types of computations integral to an undergraduate chemistry curriculum,
but they also bring many chemical principles to life for students. Not
only do students leave these courses with a valuable research skill, but
they appreciate the importance of the fundamental principles taught in
physical chemistry.
4 Analytical Chemistry
The use of computers in analytical chemistry is obvious with
respect to data acquisition. Most analytical instruments are
interfaced to computers, and data acquisition and analysis are carried
out through this interface. Spreadsheet programs are also used often
in analytical chemistry for the purpose of constructing calibration
curves and performing statistical analyses on data. However, we
suggest that computers can be used for more than developing
calibration equations. Specifically, we provide examples of how
electronic structure computations can be used to provide students with
a basic understanding of principles in chromatography and
spectroscopy, important concepts and topics in analytical
chemistry. We also remind the reader that analytical chemistry is very
much laboratory-driven, and the examples we present are employed in
conjunction with actual experiments that students perform in the
analytical laboratory.
4.1 Chromatography
As our first example, we describe how electronic structure computations
can be used effectively in developing a student's understanding of chromatographic
separations. Students learn in organic chemistry through a computational/laboratory
exercise involving TLC (described earlier) that elution order in chromatographic
separations can be predicted based on relative polarities of solutes. This
also holds true for gas and liquid chromatographic separations based on
partitioning (preferential solubility). One of the first "rules"
that students learn in general chemistry is "like dissolves like."
This is why ionic and polar compounds either dissolve or are miscible in
water (a very polar solvent) and relatively non-polar compounds (oils and
fats) do not dissolve or mix with water. Students also learn that polar
compounds have associated with them a non-zero dipole moment while non-polar
compounds possess very low dipole moments that approach zero. Based on
these simple and general principles, we can perform an analytical separation
of a mixture of several different compounds based on their relative attraction
to or solubility in a liquid stationary phase. An experiment often performed
in analytical chemistry courses is the separation of caffeine and aspirin
contained in analgesic tablets. The particular experiment we perform is
a liquid chromatographic separation that employs a polar mobile phase to
carry the solutes through a closed column. The column is packed with a
very non-polar stationary phase. Therefore, the less polar of the two solutes
will be attracted to the non-polar stationary phase and exit the column
at a later time while the more polar of the two solutes will travel with
the mobile phase and exit the column at an early time. In this particular
example, we use an electronic structure computational program to calculate
the dipole moments of the aspirin and caffeine molecules, use these calculated
dipole moments to predict their relative polarities, and thereby predict
their elution order. Our prediction can be correlated with the actual experimental
observations made in the laboratory. The results from this specific calculation
indicate that caffeine is the most polar molecule, and students predict
that it will exit the column first. However, this prediction may or may
not be accurate based on the identity and pH of the mobile phase. Specifically,
the elution order is caffeine first followed by aspirin when the pH of
the mobile phase is 3. At pH 4, however, this prediction does not fit the
experimental observations. However, this prompts students to consider in
what forms aspirin and caffeine exist at the two different pH values. Based
on initial experiences with acids and pKa values in organic
chemistry, students ultimately realize that aspirin is deprotonated (i.e.,
charged) at pH 4. The charged character of aspirin at this pH makes it
very polar, and this is enough to reverse the elution order of caffeine
and aspirin. Recalculating the dipole moment for aspirin in its deprotonated
form gives numbers (and a predicted elution order) consistent with the
experimental results.
4.2 Spectroscopy
Another important topic in analytical chemistry is spectroscopy, the
study of the interaction of light with matter. Spectroscopic analysis is
very important in analytical chemistry, and electronic structure computational
programs like PC-Spartan + can be employed as very effective tools
for teaching fundamental concepts. It is often difficult for student to
visualize what actually takes place when a molecule interacts with light.
We have employed electronic structure computational programs to aid in
their understanding of molecular absorption spectroscopy. In the case where
a molecule absorbs ultraviolet or visible light, electrons in the molecule
are promoted to higher energy levels. This is difficult for students to
visualize conceptually. However, if a student can see a picture of electron
density in a molecule (electrostatic potential) before and after it absorbs
light, his or her fundamental understanding of this process will become
more clear. Most electronic structure computational programs allow the
user to construct a molecule and calculate and visualize the ground state
or HOMO (Highest Occupied Molecular Orbital) and the excited state or LUMO
(Lowest Unoccupied Molecular Orbital). Depending on the molecule and functionalities,
one can observe differences in the electron densities of specific functional
groups that give rise to absorption signals in the ultraviolet or visible
regions of the electromagnetic spectrum.
4.3 Predicting Trends
In advanced analytical chemistry courses it is possible to enhance understanding
of spectroscopic trends by performing calculations for a particular family
of molecules. As an example, we use the family of  -diphenylpolyenes to explain the experimental observation that absorption
maxima shift to longer wavelengths when the number of conjugated double
bonds is increased. The explanation is based upon the actual energy gap
(
-diphenylpolyenes to explain the experimental observation that absorption
maxima shift to longer wavelengths when the number of conjugated double
bonds is increased. The explanation is based upon the actual energy gap
( )
between the HOMO and LUMO. An electronic structure computational program
can be used to calculate molecular orbital energies for each of the molecules
in the series. We choose trans-stilbene (tS), 1,4-diphenyl-1,3-butadiene
(DPB), 1,6-diphenyl, 1,3,5-hexatriene (DPH), and 1,8-diphenyl-1,3,5,7-octatetraene
(DPO), and perform ab initio calculations employing the 3-21G(*)
basis set. Although the absolute orbital energies may have quite a significant
error, we are merely interested in the trend exhibited by a family of similar
molecules. The trend itself is self-consistent, so it is possible to draw
some useful conclusions from the data. From these calculations, students
conclude that the HOMO energies increase as the number of conjugated double
bonds increases while the LUMO energies exhibit a systematic decrease with
increasing conjugation. The end result is that the energy gap between the
HOMO and LUMO decreases as the number of conjugated double bonds increases,
giving rise to a red shift in absorption spectra with increasing conjugation
[see Fig. 1]. )
between the HOMO and LUMO. An electronic structure computational program
can be used to calculate molecular orbital energies for each of the molecules
in the series. We choose trans-stilbene (tS), 1,4-diphenyl-1,3-butadiene
(DPB), 1,6-diphenyl, 1,3,5-hexatriene (DPH), and 1,8-diphenyl-1,3,5,7-octatetraene
(DPO), and perform ab initio calculations employing the 3-21G(*)
basis set. Although the absolute orbital energies may have quite a significant
error, we are merely interested in the trend exhibited by a family of similar
molecules. The trend itself is self-consistent, so it is possible to draw
some useful conclusions from the data. From these calculations, students
conclude that the HOMO energies increase as the number of conjugated double
bonds increases while the LUMO energies exhibit a systematic decrease with
increasing conjugation. The end result is that the energy gap between the
HOMO and LUMO decreases as the number of conjugated double bonds increases,
giving rise to a red shift in absorption spectra with increasing conjugation
[see Fig. 1].
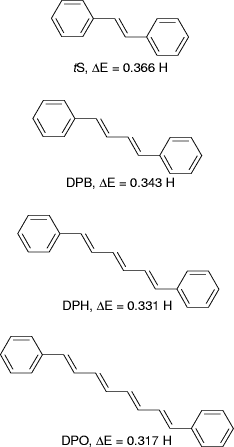
Figure 1: Structures of diphenylpolyenes and the energy difference 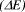 between the HOMO and LUMO in Hartrees (H).
between the HOMO and LUMO in Hartrees (H).
4.4 Vibrational Spectroscopy
At this point we wish to address briefly the applications of electronic
structure computations in advanced analytical spectroscopy courses and
their potential overlap with advanced inorganic courses. Programs like
PC-Spartan + allow the user to build molecules, calculate the fundamental
vibrational frequencies of these molecules, and animate the vibrations.
This is particularly useful in helping students understand selection rules
for vibrational spectra and their correlation with symmetry. Many programs
label each calculated frequency with symmetry notation. Students can also
use calculated vibrational frequencies to make band assignments in an experimental
spectrum. While there is a finite difference between the experimental and
calculated energies, students are expected to make use of their chemical
intuition and knowledge of symmetry as they assign the vibrational modes.
5 Conclusion
The use of electronic structure computations in the undergraduate chemistry
curriculum has endless possibilities. We have presented some of the ways
we have used this type of software in our courses; however, this is not
to suggest the limit to which this software can be used in the instruction
of these courses. We have found that the use of such software, though an
important addition to the curriculum, does not require the removal
of any significant content from these courses. These types of computations
enhance student learning through better visualization and expose students
to modern research tools in chemistry.
References
[Wavefunction, Inc.] Wavefunction, Inc.; 18401 Von
Karman, Suite 370, Irvine, CA 92715.
[Hehre et al. 1996] Hehre, W.J., Shusterman, A.J.,
Huang, W.W.: "A Laboratory Book of Computational Organic Chemistry";
Wavefunction, Inc. / Irvine (1996).
[Waterloo Maple Inc] Waterloo Maple Inc.
|