|
Query Order and the Polynomial Hierarchy
Edith Hemaspaandra (Department of Mathematics, Le Moyne College, USA)
Lane A. Hemaspaandra (Department of Computer Science, University of Rochester, USA)
Harald Hempel (Institut für Informatik, Friedrich-Schiller-Universität Jena, Germany)
Abstract: Hemaspaandra, Hempel, and Wechsung [HHW] initiated the field of query order, which studies the ways in which computational power is affected by the order in which information sources are accessed. The present paper studies, for the first time, query order as it applies to the levels of the polynomial hierarchy. 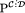 denotes the class of languages computable by a polynomial-time machine that is allowed one query to denotes the class of languages computable by a polynomial-time machine that is allowed one query to 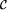 followed by one query to followed by one query to 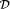 [HHW]. We prove that the levels of the polynomial hierarchy are order-oblivious: [HHW]. We prove that the levels of the polynomial hierarchy are order-oblivious: 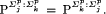 Yet, we also show that these ordered query classes form new levels in the polynomial hierarchy unless the polynomial hierarchy collapses. We prove that all leaf language classes - and thus essentially all standard complexity classes - inherit all order-obliviousness results that hold for P. Yet, we also show that these ordered query classes form new levels in the polynomial hierarchy unless the polynomial hierarchy collapses. We prove that all leaf language classes - and thus essentially all standard complexity classes - inherit all order-obliviousness results that hold for P.
Keywords: commutative queries, complexity classes, downward separation, ordered computation, polynomial hierarchy, query order
Categories: F.1.3
|