|
Componentwise Distance to Singularity
G. Rex (Institute of Mathematics, University of Leipzig, Germany)
Abstract: A perturbation matrix 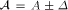 is considered, where is considered, where 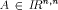 and and 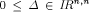 . The matrix . The matrix  is singular iff is singular iff  contains a real singular matrix. A problem is to decide if contains a real singular matrix. A problem is to decide if  is singular or nonsingular, a NP-hard problem. The decision can be made by the computation of the componentwise distance to the nearest singular matrix defined on the basis of the real spectral radius, and by the solution of 4n eigenvalue problems. is singular or nonsingular, a NP-hard problem. The decision can be made by the computation of the componentwise distance to the nearest singular matrix defined on the basis of the real spectral radius, and by the solution of 4n eigenvalue problems. Theorem 6 gives a new computation basis, a natural way to the "componentwise distance ..." definition, and a motivation to rename this in radius of singularity denoted by sir 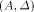 . . This new way shows: (i) - sir results from a real nonnegative eigensolution of a non-linear mapping, (ii) - sir has a norm representation, (iii) - sir can be computed by 2n-1 nonnegative eigensolutions of the nonlinear mapping, (iv) - for the special case 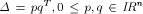 a formula for a computation of sir is given, also a trivial algorithm for the computation, and some examples as demonstration. a formula for a computation of sir is given, also a trivial algorithm for the computation, and some examples as demonstration.
Keywords: NP-hard, componentwise distance to the nearest singular matrix, interval matrix, perturbation matrix, radius of singularity
|